Finding the standard deviation

The standard deviation describes how the values are distributed over the data sample and is a measure of how far the data points deviate from the mean. It is denoted by σ.

Here are two standard deviation formulas:

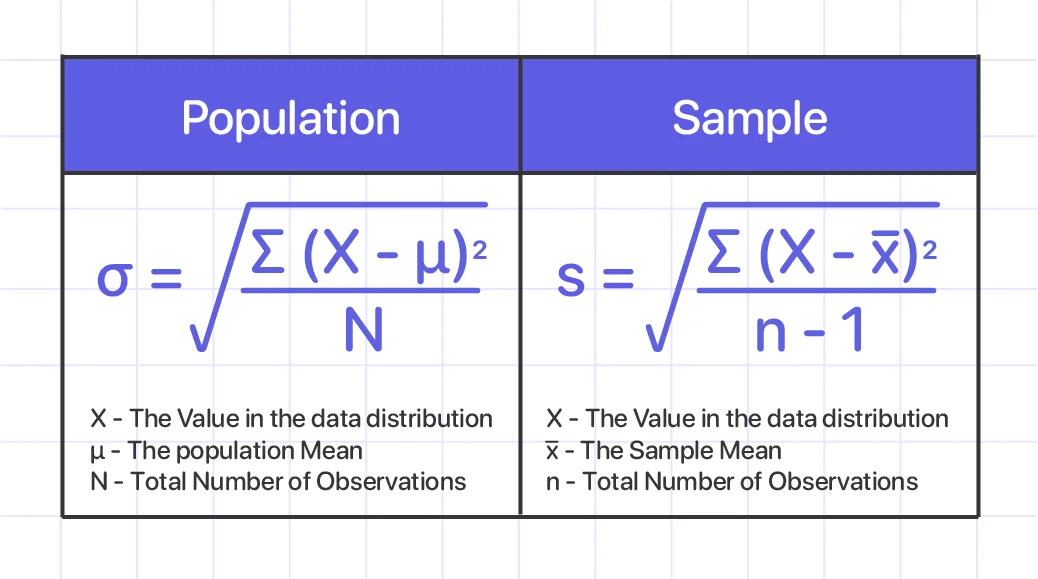
Example 1:

The garden has 39 plants. A few plants were chosen at random and their heights in centimeters were recorded as follows: 51, 38, 79, 46, and 57. Determine their heights' standard deviation.

Solution:

N = 5

Mean (x) = (51 + 38 + 79 +46 + 57) / 5 = 54.2

Standard Deviation = √((51 - 54.2)² + (38 - 54.2)² + (79 - 54.2)² + (46 - 54.2)² + (57 - 54.2)²) / 5 = 15.5

Answer: Standart deviation for this data is 15.5

Example 2:

A data set has a mean score of 40 and a standard deviation of 3. Find the z-score of the value 42.

Solution:

z = (42 - 40) / 3 = 2 / 3 = 0.67

Answer: The z-score of 42 is 0.67