MathMaster Blog

Dividing radicals implies the use of the Product and Quotient Rules.

According to the Product Rule, the product of two or more numbers raised to a power equals the product of each number raised to the same power.

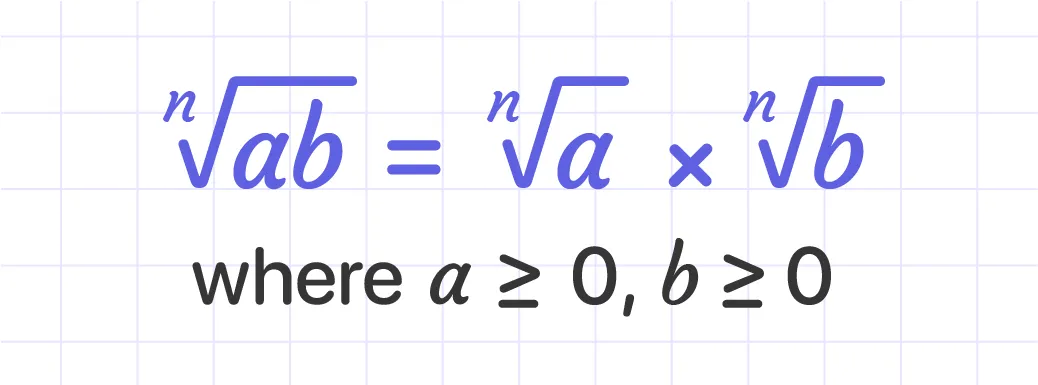
The Quotient Rule states that the radical of a quotient is equal to the quotient of the radicals of the numerator and denominator.

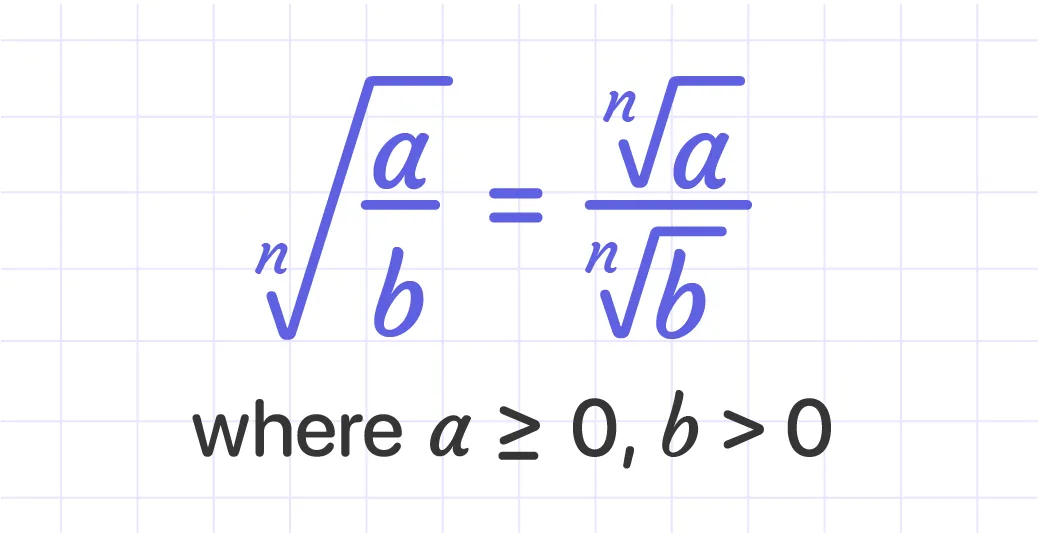
When dividing radicals (using the same index), divide under the radical first, then in front of the radical.

Example 1:

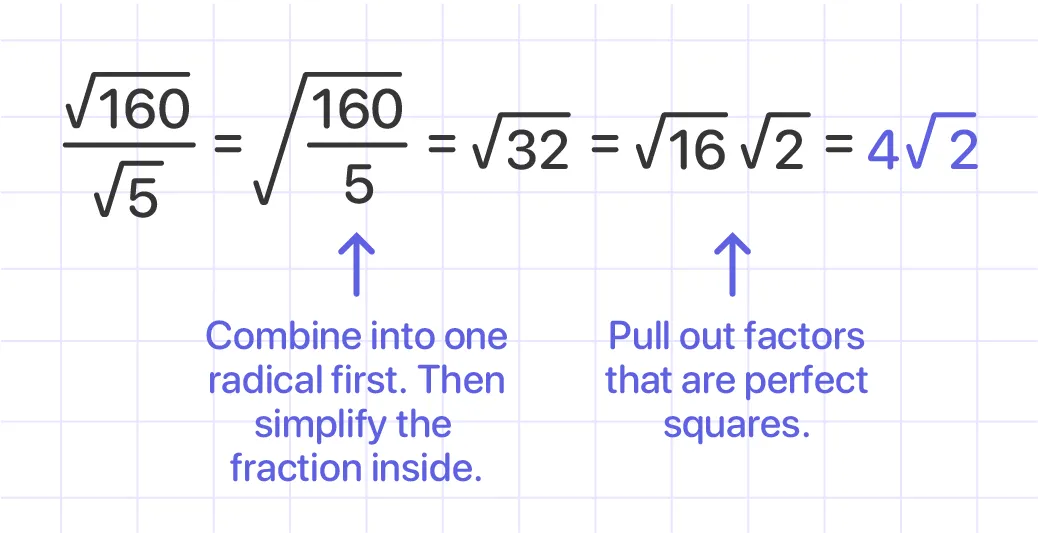
Answer: $4\sqrt{2}$

Example 2:

Divide $\frac{18\sqrt{24}}{6\sqrt{3}}$.

Solution:

Divide out front and divide under the radicals:

$\frac{18\sqrt{24}}{6\sqrt{3}} = 3\sqrt{8}$

Then simplify the result and solve.

$3\sqrt{8} = 3\sqrt{4 \cdot 2} = 2\sqrt{2} \cdot 3 = 6\sqrt{2}$

Answer: $6\sqrt{2}$