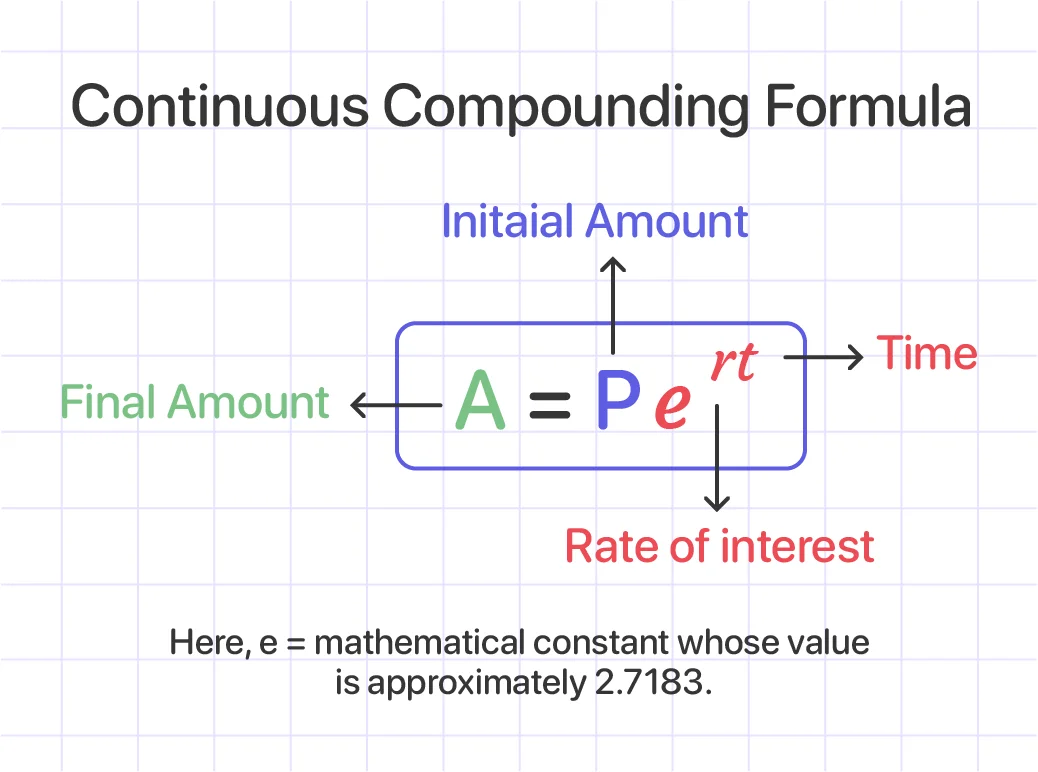
Continuous compounding

The continuous compounding formula calculates the interest earned which is continuously compounded for an infinite time period.

Example 1:

An initial amount of money is placed in an account at an interest rate of 5% per year, compounded continuously after 6 years, there is $2807.71 in the account. Find the initial amount placed in the account. Round to the nearest cent.

Solution:

Using the Continuous Compounding formula, we have:

r = 5%/100 = 0.05

t = 6 years

A = 2807.71 dollars

P x e^(rt)= A

P x e^(0.05 x 6) = 2807.71

P x e^(0.3) = 2807.71

P = 2807.71/(e^(0.3)) = 2080 dollars approximately.

Answer: $2080

Example 2:

Calculate the compounding interest on a $10,000 principal with an interest rate of 8% and a time period of one year. Compounding is done once a year, semi-annually, quarterly, monthly, and continuously.

Solution:

Annual Compounding Future Value:

• Future Value = 10,000 x (1 + 0.08)^1
• Future Value = $10,800

Semi-Annual Compounding Future Value:

• Future Value = 10,000 x (1 + 0.08/2)^2
• Future Value = 10,000 x (1.04)^2
• Future Value = 10,000 x 1.0816
• Future Value = $10,816.0

Quarterly Compounding Future Value:

• Future Value = 10,000 x (1 + 0.08/4)^4
• Future Value = 10,000 x (1.04)^4
• Future Value = 10,000 x 1.08243
• Future Value = $10,824.3

Monthly Compounding Future Value:

• Future Value = 10,000 x (1 + 0.08/12)^(12)
• Future Value = 10,000 x (1.006)^4
• Future Value = 10,000 x 1.083
• Future Value = $10,830

Continuous Compounding Future Value:

• Future Value = 10,000 x e 0.08
• Future Value = 10,000 x 1.08328
• Future Value = $10,832.87

The interest computed by continuous compounding is $832.9, which is only $2.9 higher than monthly compounding, as seen in the previous example of compounding calculations with varied frequency. In practice, it is, therefore, more practicable to use monthly or daily compounding interest rates rather than continuous compounding interest rates.